

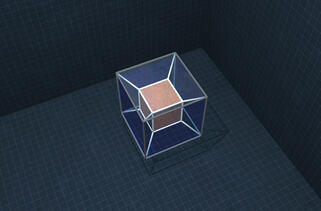


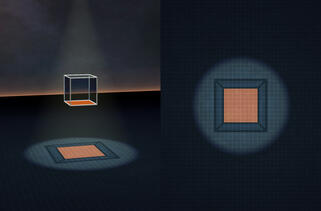
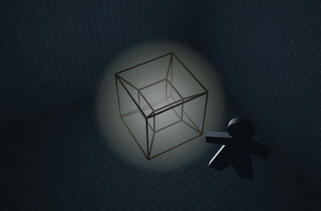
The Fourth Dimension is a 30-page interactive book that explains a single mathematical idea in language anyone can understand.
Instead of static images or canned videos, this app employs a unique 3D touch interface that lets you literally grasp the concept of the fourth dimension with your own hands.

“One of the coolest apps I’ve ever used… The Fourth Dimension is to boring learning apps what Carl Sagan’s Cosmos was to staid science documentaries.”

“Not only can you blow your own mind over and over again, you can also enjoy watching your friends’ minds being blown in front of you.”

“The app is very cool, and it’s unlike pretty much anything we’ve seen in the App Store.”
“This is one of my most favorite iOS apps ever.” — George Musser, contributing editor for Scientific American and author of The Complete Idiot’s Guide to String Theory


